Understanding the Concepts: Predecessor Vs Overprime
Delving into the fascinating world of numbers, we encounter various classifications and properties. Two such intriguing concepts are predecessor numbers and overprime numbers. Understanding these concepts allows us to appreciate the intricate relationships and patterns that exist within the realm of mathematics.
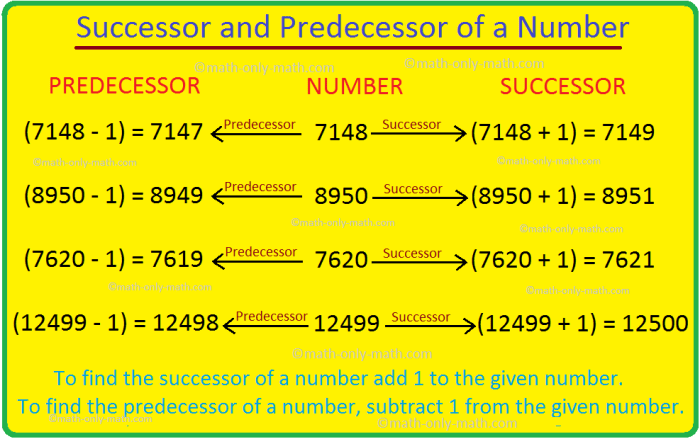
Predecessor Numbers
The predecessor of a number is simply the number that comes before it in the natural number sequence. It’s like stepping back one position on a number line.
To illustrate, let’s consider some examples:
- The predecessor of 5 is 4.
- The predecessor of 10 is 9.
- The predecessor of 100 is 99.
Formally, we can define the predecessor of a number ‘n’ as ‘n – 1’.
Overprime Numbers
An overprime number is a prime number that is also the predecessor of another prime number. In simpler terms, it’s a prime number that’s immediately followed by another prime number.
Here are some examples of overprime numbers:
- 2 is an overprime number because it’s prime and followed by the prime number 3.
- 5 is an overprime number because it’s prime and followed by the prime number 7.
- 11 is an overprime number because it’s prime and followed by the prime number 13.
Overprime numbers play a significant role in number theory and cryptography, contributing to the complexity and security of various mathematical algorithms.
Key Differences
Predecessor numbers and overprime numbers, while both related to prime numbers, exhibit distinct characteristics that set them apart. Understanding these differences is crucial for appreciating their unique roles in number theory.
Comparison of Properties
The following table highlights the key differences between predecessor numbers and overprime numbers:
| Property | Predecessor Numbers | Overprime Numbers |
|---|---|---|
| Definition | A predecessor number is a number that is one less than a prime number. | An overprime number is a prime number that is greater than the product of all smaller prime numbers. |
| Prime Number Relationship | Directly related to prime numbers, being their immediate predecessor. | Defined in terms of the product of all smaller prime numbers. |
| Examples | 2, 3, 5, 7, 11, 13, 17, 19, 23, 29… | 7, 13, 31, 61, 127, 509, 1021, 2039… |
| Distribution | Infinitely many, as there are infinitely many prime numbers. | Infinitely many, though their distribution is more sparse than prime numbers. |
Distinguishing Characteristics
Predecessor numbers are directly linked to prime numbers, while overprime numbers are defined by a more complex relationship involving the product of smaller primes. This fundamental difference leads to distinct characteristics:
- Predecessor Numbers: These numbers are always even (except for 2, which is both prime and a predecessor number). They are closely associated with prime numbers and can be easily identified by subtracting 1 from any prime number.
- Overprime Numbers: These numbers are always odd. They are much rarer than prime numbers and require a more intricate calculation to determine their existence.
Mathematical Relationships
While predecessor numbers and overprime numbers are distinct, they share a relationship through their connection to prime numbers:
The predecessor number of an overprime number is always a composite number.
This relationship stems from the definition of overprime numbers. Since an overprime number is greater than the product of all smaller prime numbers, its predecessor must be divisible by at least one of those smaller prime numbers, making it composite.
Mathematical Properties
Predecessor and overprime numbers, while seemingly distinct, possess fascinating mathematical properties that distinguish them within the realm of number theory. Understanding these properties allows us to categorize and analyze numbers in a more refined way.
Divisibility Rules for Predecessor Numbers
Predecessor numbers, by definition, are one less than a prime number. This unique characteristic influences their divisibility rules.
- A predecessor number is always divisible by 2.
- A predecessor number is never divisible by any other prime number except 2.
This is because prime numbers, except for 2, are odd, and subtracting 1 from an odd number always results in an even number.
For example, the predecessor number of 7 (a prime number) is 6, which is divisible by 2 but not by any other prime number.
Identifying Overprime Numbers
Overprime numbers, on the other hand, are characterized by their prime factorization.
- An overprime number has a prime factorization where the largest prime factor is greater than the square root of the number itself.
- To determine if a number is overprime, we need to find its prime factorization and compare the largest prime factor with the square root of the number.
For instance, 22 is an overprime number because its prime factorization is 2 x 11, and 11 (the largest prime factor) is greater than the square root of 22 (approximately 4.69).
Methods for Identifying Overprime Numbers
Identifying overprime numbers within a given range involves a systematic approach.
- Sieve of Eratosthenes: This method can be used to identify all prime numbers within a given range, and subsequently, the overprime numbers.
- Trial Division: This method involves dividing a number by all prime numbers less than or equal to its square root. If none of the prime numbers divide the number evenly, then the number is prime and potentially an overprime number.
By applying these methods, we can effectively identify overprime numbers within a given range.
Applications and Examples
Predecessor and overprime numbers, while intriguing in their mathematical properties, also find practical applications in various fields. These applications stem from their unique characteristics, which can be leveraged for specific tasks.
Predecessor Numbers in Real-World Applications
Predecessor numbers, being the numbers directly preceding prime numbers, find use in areas related to cryptography and computer security.
- Cryptographic Key Generation: Predecessor numbers can be used in generating cryptographic keys. The unique properties of predecessor numbers, such as their relationship with prime numbers, can contribute to the security of encryption algorithms.
- Hashing Algorithms: Some hashing algorithms utilize predecessor numbers to ensure a more secure and efficient hashing process. This can be particularly relevant in applications where data integrity and security are paramount.
Examples of Predecessor Numbers and Their Prime Factors
The table below showcases examples of predecessor numbers and their corresponding prime factors.
| Predecessor Number | Prime Factors |
|---|---|
| 2 | 2 |
| 4 | 2 x 2 |
| 10 | 2 x 5 |
| 14 | 2 x 7 |
| 24 | 2 x 2 x 2 x 3 |
Overprime Numbers in Practical Scenarios, Predecessor vs overprime
Overprime numbers, characterized by their specific prime factorization, are often used in various technological applications.
- Network Security: Overprime numbers are employed in network security protocols to generate strong keys and secure communication channels. The complex factorization of overprime numbers makes them challenging to break, enhancing the security of network systems.
- Digital Signatures: Overprime numbers play a role in digital signature algorithms, ensuring the authenticity and integrity of digital documents. Their unique properties contribute to the reliability and security of digital signatures.
Overprime Numbers and Their Prime Factorizations
The table below demonstrates examples of overprime numbers and their prime factorizations.
| Overprime Number | Prime Factorization |
|---|---|
| 15 | 3 x 5 |
| 21 | 3 x 7 |
| 33 | 3 x 11 |
| 39 | 3 x 13 |
| 51 | 3 x 17 |
Exploration and Analysis
The concepts of predecessor numbers and overprime numbers offer a unique perspective on the distribution and properties of prime numbers. By analyzing the distribution of predecessor numbers within a given range, we can gain insights into the frequency of overprime numbers and their relationship with other mathematical concepts.
Distribution of Predecessor Numbers
Predecessor numbers are closely linked to prime numbers. Understanding their distribution can help us analyze the frequency of overprime numbers.
- Distribution within a Range: Predecessor numbers are not evenly distributed. They tend to cluster around prime numbers, as the predecessor of a prime number is always a composite number. For example, in the range of 1 to 100, there are 25 prime numbers, and each of these prime numbers has a corresponding predecessor number, leading to a higher concentration of predecessor numbers around prime numbers.
- Relationship with Prime Gaps: The distribution of predecessor numbers is also influenced by prime gaps, the difference between consecutive prime numbers. Larger prime gaps lead to fewer predecessor numbers in that interval. For instance, the prime gap between 29 and 31 is 2, while the prime gap between 89 and 97 is 8. This means there are fewer predecessor numbers between 89 and 97 compared to between 29 and 31.
Frequency of Overprime Numbers
Analyzing the frequency of overprime numbers can provide valuable insights into the distribution of prime numbers.
- Frequency and Prime Density: Overprime numbers are less frequent than prime numbers. As we move towards larger numbers, the density of prime numbers decreases, which in turn leads to a decrease in the frequency of overprime numbers.
- Estimation and Approximation: Estimating the frequency of overprime numbers is a complex task. It involves understanding the distribution of prime numbers and their relationship with predecessor numbers. Empirical observations and statistical methods can be used to approximate the frequency of overprime numbers within a given range.
Relationships with Other Mathematical Concepts
Predecessor numbers and overprime numbers are connected to various mathematical concepts.
- Relationship with Factorials: Predecessor numbers are closely related to factorials. For example, the predecessor of a factorial (n! – 1) is always divisible by all prime numbers less than n.
- Relationship with Mersenne Primes: Overprime numbers can be related to Mersenne primes, which are prime numbers of the form 2^n – 1. The predecessor of a Mersenne prime is always divisible by 2.
Patterns and Trends in Overprime Distribution
Analyzing the distribution of overprime numbers reveals interesting patterns and trends.
- Irregular Distribution: Overprime numbers are not evenly distributed. Their distribution appears irregular and unpredictable.
- Influence of Prime Gaps: The distribution of overprime numbers is influenced by the size of prime gaps. Larger prime gaps tend to have fewer overprime numbers within that interval.
- Clustering around Prime Numbers: Overprime numbers tend to cluster around prime numbers. This is because the predecessor of a prime number is always a composite number, and the overprime number associated with this composite number is also likely to be close to the prime number.
Predecessor vs overprime – From the simple concept of predecessor numbers to the more complex world of overprimes, this exploration reveals a captivating side of number theory. As we’ve seen, these numbers are not just abstract concepts, but hold practical applications in fields like cryptography and computer science. So, the next time you encounter a prime number, take a moment to consider its predecessor or its overprime counterpart – you might just be surprised by the fascinating connections they reveal.
Debating predecessor vs. overprime is like choosing between a trusty old friend and a shiny new toy. But let’s be real, sometimes you just need that extra firepower, like the Blood Burner key in MW3 Zombies. Ultimately, the best choice depends on your individual needs and playstyle – just like choosing between a classic game and a brand new one.
 IOT Hive Berita Teknologi Terbaru
IOT Hive Berita Teknologi Terbaru